| Issue |
ESAIM: M2AN
Volume 44, Number 1, January-February 2010
|
|
|---|---|---|
| Page(s) | 75 - 108 | |
| DOI | https://doi.org/10.1051/m2an/2009041 | |
| Published online | 03 November 2009 | |
Weighted regularization for composite materials in electromagnetism
1
Laboratoire POEMS, UMR 7231 CNRS/ENSTA/INRIA, ENSTA ParisTech, 32 boulevard Victor, 75739 Paris Cedex 15, France. Patrick.Ciarlet@ensta.fr
2
Laboratoire de Mathématiques, FRE 3111, UFR Sciences exactes et naturelles, Université de Reims Champagne-Ardenne, Moulin de la Housse – B.P. 1039, 51687 Reims Cedex 2, France. francois.lefevre@univ-reims.fr; stephanie.lohrengel@univ-reims.fr
3
LAMAV, Université de Valenciennes et du Hainaut Cambrésis, Le Mont Houy, 59313 Valenciennes Cedex 9, France. serge.nicaise@univ-valenciennes.fr
Received:
10
September
2008
In this paper, a weighted regularization method for the time-harmonic Maxwell equations
with perfect conducting or impedance boundary condition in composite materials is presented.
The computational domain Ω is the union
of polygonal or polyhedral subdomains made of different materials. As a result, the electromagnetic field presents singularities
near geometric singularities, which are the interior and exterior edges and corners. The variational formulation of the
weighted regularized problem is given on the subspace of
 (
( ;Ω)
whose fields
;Ω)
whose fields  satisfy
satisfy  div (
div ( )∈ L2(Ω) and have vanishing tangential trace
or tangential trace in L2(
)∈ L2(Ω) and have vanishing tangential trace
or tangential trace in L2( ). The weight function
). The weight function 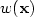 is equivalent
to the distance of
is equivalent
to the distance of  to the geometric singularities and the minimal weight parameter α
is given in terms of the singular exponents of a scalar transmission problem.
A density result is proven that guarantees the approximability of the solution field by piecewise regular fields.
Numerical results for the discretization of the source problem
by means of Lagrange Finite Elements of type P1 and P2 are given on
uniform and appropriately refined two-dimensional meshes.
The performance of the method in the case of eigenvalue problems is addressed.
to the geometric singularities and the minimal weight parameter α
is given in terms of the singular exponents of a scalar transmission problem.
A density result is proven that guarantees the approximability of the solution field by piecewise regular fields.
Numerical results for the discretization of the source problem
by means of Lagrange Finite Elements of type P1 and P2 are given on
uniform and appropriately refined two-dimensional meshes.
The performance of the method in the case of eigenvalue problems is addressed.
Mathematics Subject Classification: 78M10 / 65N30 / 78A48
Key words: Maxwell's equations / interface problem / singularities of solutions / density results / weighted regularization
© EDP Sciences, SMAI, 2009
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.


