EDP Sciences logo
Subscriber Authentication Point
Search
Menu
All issues Volume 55 / No 3 (May-June 2021) ESAIM: M2AN, 55 3 (2021) 789-805 References
Free Access
Issue
ESAIM: M2AN
Volume 55, Number 3, May-June 2021
Page(s) 789 - 805
DOI https://doi.org/10.1051/m2an/2020087
Published online 05 May 2021
  1. G. Allaire, Homogenization of the Stokes flow in a connected porous medium. Asymptotic Anal. 2 (1989) 203–222. [Google Scholar]
  2. G. Allaire, Homogenization and two-scale convergence. SIAM J. Math. Anal. 23 (1992) 1482–1518. [Google Scholar]
  3. G.I. Barenblatt, Y.P. Zheltov and I.N. Kochina, On basic conceptions of the theory of homogeneous fluids seepage in fractured rocks (in Russian). Prikl. Mat. i Mekh. 24 (1960) 852–864. [Google Scholar]
  4. G.I. Barenblatt, V.M. Entov and V.M. Ryzhik, Theory of Fluid Flows Through Natural Rocks. Kluwer Acad. Pub., Dordrecht (1990). [Google Scholar]
  5. G.S. Beavers and D.D. Joseph, Boundary conditions at a naturally permeable wall. J. Fluid Mech. 30 (1967) 197–207. [Google Scholar]
  6. D. Cioranescu and J. Saint-Jean-Paulin, Homogenization in open sets with holes. J. Math. Anal. Appl. 71 (1979) 590–607. [CrossRef] [MathSciNet] [Google Scholar]
  7. D. Cioranescu and P. Donato, An introduction to homogenization. In: Vol. 17 of Oxford Lecture Series in Mathematics and Its Applications. Oxford Univ. Press, New York (1999). [Google Scholar]
  8. H.I. Ene and D. Poliševski, Thermal Flow in Porous Media. D. Reidel Pub. Co., Dordrecht (1987). [Google Scholar]
  9. H.I. Ene and D. Poliševski, Model of diffusion in partially fissured media. ZAMP 53 (2002) 1052–1059. [Google Scholar]
  10. P. Forchheimer, Wasserbewegung durch boden. Z. Ver. Dtsch. Ing. 45 (1901) 1782–1788. [Google Scholar]
  11. R. Glowinski and A. Marrocco, Sur l’approximation, par éléments finis d’ordre un, et la résolution, par pénalisation-dualité, d’une classe de problèmes de Dirichlet non linéaires. Rev. Française Automat. Informat. Recherche Opérationnelle, Sér. Rouge Anal. Numér. 9 (1975) 41–76. [Google Scholar]
  12. I. Gruais and D. Poliševski, Fluid flows through fractured porous media along Beavers-Joseph interfaces. J. Math. Pures Appl. 102 (2014) 482–497. [Google Scholar]
  13. I. Gruais and D. Poliševski, Heat transfer models for two-component media with interfacial jump. Appl. Anal. 96 (2017) 247–260. [Google Scholar]
  14. I. Gruais and D. Poliševski, Model of two-temperature convective transfer in porous media. Z. Angew. Math. Phys. 68 (2017) 11. [Google Scholar]
  15. W. Jäger and A. Mikelić, Modeling effective interface laws for transport phenomena between an unconfined fluid and a porous medium using homogenization. Transp. Porous Med. 78 (2009) 489–508. [Google Scholar]
  16. I.P. Jones, Low Reynolds number flow past a porous spherical shell. Proc Camb. Phil. Soc. 73 (1973) 231–238. [Google Scholar]
  17. D. Kinderlehrer and G. Stampacchia, An introduction to Variational Inequalities and Their Applications. Academic Press, New-York (1980). [Google Scholar]
  18. R. Lipton and M. Avellaneda, Darcy’s law for slow viscous flow past a stationary array of bubbles. Proc. Roy. Soc. Edinburgh Sect. A 114 (1990) 71–79. [Google Scholar]
  19. D. Lukkassen, G. Nguetseng and P. Wall, Two-scale convergence. Int. J. Pure Appl. Math. 2 (2002) 35–86. [Google Scholar]
  20. V.P. Mikhailov, Partial Differential Equations. Mir Publishers, Moscow (1978). [Google Scholar]
  21. M. Muskat, The Flow of Homogeneous Fluids through Porous Media. Edwards, MI (1946). [Google Scholar]
  22. G. Nguetseng, A general convergence result for a functional related to the theory of homogenization. SIAM J. Math. Anal. 20 (1989) 608–623. [CrossRef] [MathSciNet] [Google Scholar]
  23. D. Poliševski, On the homogenization of fluid flows through periodic media. Rend. Sem. Mat. Univers. Politecn. Torino 45 (1987) 129–139. [Google Scholar]
  24. D. Poliševski, Basic homogenization results for a biconnected ε-periodic structure. Appl. Anal. 82 (2003) 301–309. [Google Scholar]
  25. D. Poliševski, The regularized diffusion in partially fractured porous media. In: Vol. 2 of Current Topics in Continuum Mechanics. Ed. Academiei, Bucharest (2003). [Google Scholar]
  26. P.G. Saffman, On the boundary condition at the interface of a porous medium. Stud. Appl. Math. 50 (1971) 93–101. [Google Scholar]
  27. R.E. Showalter and N.J. Walkington, Micro-structure models of diffusion in fissured media. J. Math. Anal. Appl. 155 (1991) 1–20. [Google Scholar]
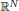  28. J. Simon, Régularité de la solution d’une équation non linéaire dans Formula . Journées d’Analyse non linéaire. Proceedings, Besançon, France, 1977. In: Vol. 665 of Lecture Notes in Mathematics. Springer-Verlag, Berlin (1978) 205–227. [Google Scholar]
  29. S. Whitaker, Flow in porous media I: A theoretical derivation of Darcy’s law. Transp. Por. Med. 1 (1986) 3–25. [Google Scholar]
  30. E. Zeidler, Nonlinear Functional Analysis and Its Applications. I. Fixed-Point Theorems. Springer-Verlag, New York (1986). [Google Scholar]

Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.

Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.

Initial download of the metrics may take a while.

Recommended for you